JAVA条件运算符
本文共 521 字,大约阅读时间需要 1 分钟。
笔者随意些的一篇小文章访问居然挺高,但是看起来有点简陋,在补充一些小图方便理解,可能是不知道条件运算符是什么有点忘了,看到三目,三元就知道了.
条件运算符(?:)
条件运算符也被称为三元运算符。该运算符有3个操作数,并且需要判断布尔表达式的值。该运算符的主要是决定哪个值应该赋值给变量。
variable x = (expression) ? value if true : value if false
代码:
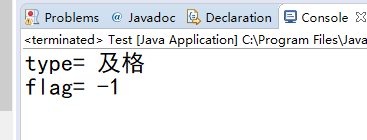
public class Test {//公共测试类,与文件名相同 public static void main(String[] args){//main程序入口 int score = 80; int x = -100; String type =score<60?"不及格":"及格"; int flag = x > 0 ? 1 : (x == 0 ? 0 : -1); System.out.println("type= " + type); System.out.println("flag= "+ flag);}} 运行效果:
转载地址:http://szzr.baihongyu.com/
你可能感兴趣的文章
MySQL分组函数
查看>>
MySQL分组查询
查看>>
Mysql分表后同结构不同名称表之间复制数据以及Update语句只更新日期加减不更改时间
查看>>
mySql分页Iimit优化
查看>>
MySQL分页查询
查看>>
mysql列转行函数是什么
查看>>
mysql创建函数报错_mysql在创建存储函数时报错
查看>>
mysql创建数据库和用户 并授权
查看>>
mysql创建数据库指定字符集
查看>>
MySql创建数据表
查看>>
MySQL创建新用户以及ERROR 1396 (HY000)问题解决
查看>>
MySQL创建用户与授权
查看>>
MySQL创建用户报错:ERROR 1396 (HY000): Operation CREATE USER failed for 'slave'@'%'
查看>>
MySQL创建索引时提示“Specified key was too long; max key length is 767 bytes”
查看>>
mysql初始密码错误问题
查看>>
MySQL删除数据几种情况以及是否释放磁盘空间【转】
查看>>
Mysql删除重复数据通用SQL
查看>>
mysql判断某一张表是否存在的sql语句以及方法
查看>>
mysql加入安装策略_一键安装mysql5.7及密码策略修改方法
查看>>
mysql加强(1)~用户权限介绍、分别使用客户端工具和命令来创建用户和分配权限
查看>>
